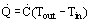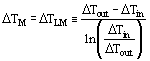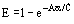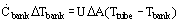Tube banks are employed in a wide range of heat exchangers. The average heat flux,
 , is expressed as a linear function of the mean temperature difference between the bulk of the fluid in the tube bank and the wall, according to a rate equation
, is expressed as a linear function of the mean temperature difference between the bulk of the fluid in the tube bank and the wall, according to a rate equation
where
 is the rate of heat transfer,
is the rate of heat transfer,
 is an overall heat transfer coefficient, and ΔTM is some representative temperature difference between the tube wall and the bulk of the fluid. A is the total heat transfer area, which for plain tubes is given by
is an overall heat transfer coefficient, and ΔTM is some representative temperature difference between the tube wall and the bulk of the fluid. A is the total heat transfer area, which for plain tubes is given by

where N is the number of tubes of outer diameter D and length L. The overall heat transfer coefficient,
 is nondimensionalized in terms of an average Nusselt Number,
is nondimensionalized in terms of an average Nusselt Number,
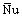 , according to
, according to

where λ is the fluid thermal conductivity, or alternatively as an average Stanton Number,
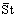 ,
,

where cp is the fluid specific heat.
In tube banks,
 is frequently correlated according to
is frequently correlated according to
where Reynolds number, Re, is defined by

umax is the bulk velocity in the minimum cross section, ρ and η are the fluid density and viscosity respectively. The Prandtl number, Pr, is just,

where cp is the specific heat, and λ is thermal conductivity. The rate of heat transfer may also be expressed in terms of an energy balance, which for single-phase heat transfer may be written,
where Tin and Tout are the bulk temperatures at the inlet and outlet of the bank, and
 is the thermal capacitance of the fluid, presumed constant over the range of interest
is the thermal capacitance of the fluid, presumed constant over the range of interest
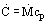
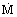 is the total mass flow rate through all of the passages in the tube bank, and cp is averaged over the range of interest.
is the total mass flow rate through all of the passages in the tube bank, and cp is averaged over the range of interest.
A local heat transfer coefficient may be defined in a similar fashion to Equation 1. The local Nu is a function of a number of parameters such bank type and geometry, flow Re, pressure gradient, location within bank etc. Figure 1 shows local Nu distributions in the interior of tube banks as a function of the angle φ measured from the front of the cylinder. It can be seen that the staggered-geometry Nu-distribution is similar to the single cylinder case, with a maximum occurring at φ = 0°. For the in-line case, Nu rises to a maximum at the reattachment point around 45°. It can also be seen that heat transfer is somewhat higher for both in-line and staggered tube banks, than for single cylinders. This is due to increased free-stream turbulence (as a result of proceeding rows) and shear, due to constriction of the flow passages. For most applications the engineer is not concerned with the details of local heat transfer in tube banks and the reader is referred to Žukauskas and Ulinskas (1988) for further information on the subject.
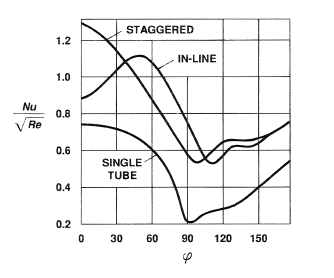
Figure 1. Variation of local heat transfer around 1) a single tube, 2) a tube in a staggered bank, 3) a tube in an in-line banks. From Žukauskas and Ulinskas (1988).
Two major sources of data on both fluid flow and heat transfer are the Delaware group in the USA and the group in the Republic of Lithuania. The work at the University of Delaware on overall heat transfer in tube banks has formed the basis for heat exchanger calculations for many years while the recent book by Žukauskas et al. (1988) is a comprehensive reference on heat transfer in tube banks based on many years of research. It contains extensive information on local and overall heat transfer. It appears that the Delaware data was gathered at nominally constant wall temperature, Tw, conditions and that of the Lithuanian group at constant wall heat flux,
 . Numerous other data also exist, in addition to these two main sources.
. Numerous other data also exist, in addition to these two main sources.
A number of empirical correlations may also be found. The Engineering Science and Data Unit correlate
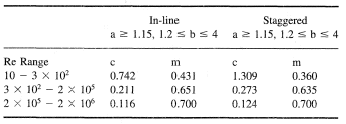
 vs. Re according to Equation (5) [ESDU (1978)]. Values of the coefficients c and m are given in Table 1 Re is based on umax (see Tube Banks, Cross Flow Over.) The correlation of Žukauskas and Ulinskas (1988) agrees well with experimental data, but exhibits poor continuity across certain Re ranges. Several other correlations may be found in the literature. Agreement among heat transfer correlations is generally better than for pressure drop correlations. In most correlations,
vs. Re according to Equation (5) [ESDU (1978)]. Values of the coefficients c and m are given in Table 1 Re is based on umax (see Tube Banks, Cross Flow Over.) The correlation of Žukauskas and Ulinskas (1988) agrees well with experimental data, but exhibits poor continuity across certain Re ranges. Several other correlations may be found in the literature. Agreement among heat transfer correlations is generally better than for pressure drop correlations. In most correlations,
 is treated as being primarily a function of Re and configuration (i.e., the pitch-to-diameter ratios are often treated as being relatively unimportant over a wide range). Similarly, the effect of thermal boundary conditions (constant Tw vs. constant
is treated as being primarily a function of Re and configuration (i.e., the pitch-to-diameter ratios are often treated as being relatively unimportant over a wide range). Similarly, the effect of thermal boundary conditions (constant Tw vs. constant
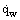 ), though often significant, is usually simply ignored.
), though often significant, is usually simply ignored.
Table 1. Coefficients used to calculate overall heat transfer in tube banks using Eq. (5) with n = 0.34, p = 0.26. From ESDU International plc (1973)
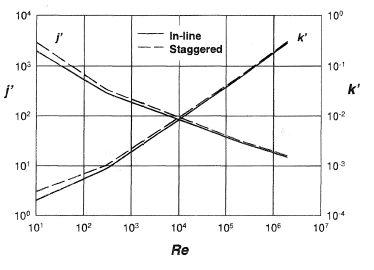
Tradition is to plot
 or
or
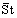 in the form of log-log plots with both the Pr-dependence and the property-variation being removed by defining
in the form of log-log plots with both the Pr-dependence and the property-variation being removed by defining


The heat-transfer factors j, k (unprimed) are Pr-independent. Primed values j', k' indicate that the influence of property variations across the boundary layer has also been taken into consideration. The Delaware group chose n = 1/3, i.e., j is the so-called Colburn heat transfer factor. ESDU (1973) and Žukauskas and Ulinskas (1988) propose similar, but slightly higher, values n = 0.34 and 0.36, respectively (over most of the range). Figure 2 shows j and k as a function of Re for Pr = 1. It can be seen that heat transfer is a little higher for staggered than in-line geometries at lower Re.
The influence of fluid properties is accounted for in Equation 5 by the term (Prw/Pr)p, where Pr is evaluated at the mean bulk temperature, and Prw at Tww. The Delaware group use (ηw/η)p, the matter is largely one of preference (but their value of p = 0.14 was based on the Seider-Tate correlation for flow inside tubes). ESDU (1973) suggest p = 0.26 regardless of whether the fluid is being heated or cooled.
The choice of reference temperature is a matter for concern since in most situations, the engineer must use a mean value for thermal design/analysis. For constant Tw the appropriate temperature to use in Eq. (1) is the log-mean temperature difference,
where ΔTin = Tin – Tw and ΔTout = Tout − Tw are the differences between the fluid and wall temperatures at the inlet and outlet, respectively (see also Mean Temperature Difference.) The reference temperature, TM, for ennumerating property values should be Tw + ΔTLM. For constant
 the arithmetic-mean temperature should be used,
the arithmetic-mean temperature should be used,

For most applications it is immaterial whether properties, ρ, η etc., are evaluated at the log-mean or the arithmetic-mean temperature, and the latter is often employed for convenience. For large numbers of banks and low values of the product Re.Pr the choice of TM is quite important.
Empirical correlations are obtained from data for idealized tube banks. In practice, Nu is a function of a number of other parameters which are often accounted for by writing,
where
 is the idealized-correlation-based Nusselt number, and the k-coefficients account for deviations from this situation.
is the idealized-correlation-based Nusselt number, and the k-coefficients account for deviations from this situation.
One example, already mentioned, is the factor (Prw/Pr)p. Other factors could include the effects of finite numbers of rows, angle of attack, or other considerations. These are discussed below.
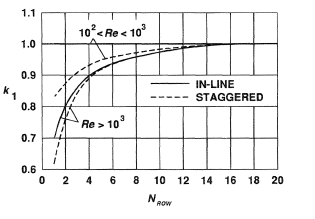
Idealized correlations, described above, are for deep tube banks, where the number of rows, Nrow, is large. For each of the first few rows in a tube bank, heat transfer may be substantially different (usually less) than occurs deep in the bank. Figure 3 shows the correction factor k1, as a function of Nrow.
In many situations, the flow is not one of pure crossflow, i.e., β < 90°. Heat transfer is reduced from by a factor k2. Figure 4 shows k2 vs. β.
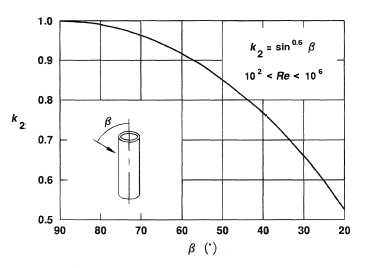
Figure 4. Influence of number of rows on overall heat transfer in tube banks. From Žukauskas and Ulinskas (1988).
Two commonly-employed methods of enhancing heat transfer in tube banks are the use of rough surfaces and finned tubes (see Augmentation of Heat Transfer.)
Rough tubes are used to increase the ratio of heat transfer to drag by increasing turbulence. The effects appear to be more pronounced for staggered than in-line geometries. Žukauskas and Ulinskas (1988) propose the following prescription for staggered geometries:


where k is the roughness height.
External fins may be employed with gas crossflow, in order to increase the heat-transfer area (internal fins are also used sometimes). The book by Stasiulevičius and Skrinska (1988) is devoted to the subject, as is a chapter in Žukauskas et al. (1988). When using finned tubes in a design, the engineer must use Nu-correlations appropriate to finned tubes, and properly account for temperature variations throughout the fin (see also Extended Surface Heat Transfer.)
A number of general purpose correlations for finned tube-banks may be found:
Gnielinski et al. in the Heat Exchanger Design Handbook (1983), ESDU (1984, 1986),
Stasiulevičius and Skrinska (1988), and Žukauskas and Ulinskas (1988)
These typically assume forms similar to Equation (5), but with additional parameters to account for the fin geometry. The engineer should obtain Nu correlations specific to the particular geometrical configuration under consideration, whenever possible.
When variation in temperature across the fins is significant, the rate equation, Equation (1), is rewritten as
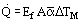
A is the total area for heat transfer, A = Af + Aw, where Af is the total area of the fins and Aw that of the exposed tube-wall. Ef is a surface effectiveness factor,

and ηf is a fin efficiency, i.e., the ratio of the actual rate of heat transfer through the fins to that which would occur if all fin material were at constant Tw.
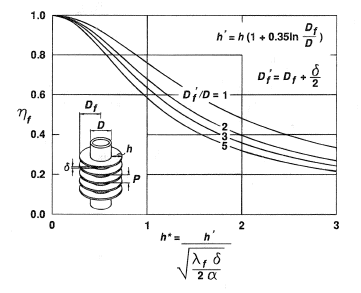
Figure 5 is a plot of hf vs. nondimensional height h*, at various diameter ratios,
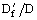 . λf is the thermal conductivity of the fin, δ the fin thickness and
. λf is the thermal conductivity of the fin, δ the fin thickness and
 the average heat transfer coefficient. Primed values,
h'
and
D'
, indicate that they are adjusted for heat transfer through the fin-tip, as indicated in Figure 5. Charts of ηf vs. h* have been devised for a variety of geometries; the article by Gnielinski et al. in the Heat Exchanger Design Handbook (1983) contains a selection. These should be used in preference to Figure 5, which was obtained from the one dimensional fin equation solution,
the average heat transfer coefficient. Primed values,
h'
and
D'
, indicate that they are adjusted for heat transfer through the fin-tip, as indicated in Figure 5. Charts of ηf vs. h* have been devised for a variety of geometries; the article by Gnielinski et al. in the Heat Exchanger Design Handbook (1983) contains a selection. These should be used in preference to Figure 5, which was obtained from the one dimensional fin equation solution,

where h' is calculated according to Schmidt [Stasiulevičlus and Skrinska (1988)].
Bypass streams are relatively ineffective in transferring heat, and are usually treated as adiabatic, i.e., only the main flow stream is used to compute heat transfer. Since the temperature and hence the viscosity differs from that in the main flow lanes, this must be taken into consideration when computing the bypass and main mass-flow rates.
How to compute the performance of a tube bank will depend to a larger extent on which of the parameters,
 ,
,
 , A, Tin, Tout,
Ċ
etc., are prescribed or required, whether fluid properties are constant, and the precision required. It is common to differentiate between "rating" and "sizing" of heat exchangers. This section is intended to provide some guidelines for the reader, not a single general-purpose procedure. Two cases will be considered in detail: plain tubes at constant Tw and non-isothermal plain or finned tubes:
, A, Tin, Tout,
Ċ
etc., are prescribed or required, whether fluid properties are constant, and the precision required. It is common to differentiate between "rating" and "sizing" of heat exchangers. This section is intended to provide some guidelines for the reader, not a single general-purpose procedure. Two cases will be considered in detail: plain tubes at constant Tw and non-isothermal plain or finned tubes:
The log-mean temperature-difference (LMTD) approach is based on the fundamental assumption that the heat flux is directly proportional to the temperature difference between the bulk of the fluid at some interior location and the wall, i.e., the rate equation, Equation 1, with ΔTM prescribed according to Equation (12).
Unless all temperatures are known, a priori, a trial-and-error method based on successive applications of the rate equation combined with a heat balance is required. An alternative approach, due to Nusselt, is to postulate the rate of heat transfer to be proportional to the difference between the inlet and wall temperatures,
The effectiveness, E, represents the ratio of the actual to the maximum possible heat transfer. For constant Tw
where the quantity A
 /C is known as the Number of Transfer Units, NTU. The advantage of Tin as reference (if known) is that no iteration is required provided fluid properties are constant and upstream values can be used. Even if these vary, the E-NTU-method is often preferable, as TM affects the calculation of
/C is known as the Number of Transfer Units, NTU. The advantage of Tin as reference (if known) is that no iteration is required provided fluid properties are constant and upstream values can be used. Even if these vary, the E-NTU-method is often preferable, as TM affects the calculation of
 via property variations only. A typical procedure is as follows:
via property variations only. A typical procedure is as follows:
Given Tin guess a value for Tout (unless known).
Calculate Re and Pr based on property values evaluated at the arithmetic-mean bulk temperature, and Prw at Tw.
Compute the value of
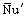 for an ideal bank using Equation (5) applying corrections, k1, k2 etc. as discussed above, to obtain
for an ideal bank using Equation (5) applying corrections, k1, k2 etc. as discussed above, to obtain
 and hence
and hence
 for the actual bank, Equation (14).
for the actual bank, Equation (14).
Compute the overall rate of heat transfer,
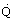 , using one of two methods: either calculate ΔTLM from Equation (12), and use the rate equation (1) to obtain
, using one of two methods: either calculate ΔTLM from Equation (12), and use the rate equation (1) to obtain
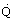 , or calculate E from
, or calculate E from
 using Equation (20) and obtain
using Equation (20) and obtain
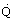 using Equation (19).
using Equation (19).
Compute the exit bulk temperature, Tout from
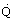 , by means of a heat balance, Equation (8).
, by means of a heat balance, Equation (8).
Reiterate steps 2–6 based on the new value of Tout (unless prescribed) until satisfactory convergence is obtained.
If changes in temperature are large, break the bank up into an individual or groups of rows, and proceed in a sequential fashion from the inlet to the exit.
In many practical engineering applications, the tube-side fluid also undergoes significant changes in temperature. Under these circumstances, Equation (1) is no longer appropriate, and the rate of heat transfer is

where U, the Overall Heat Transfer Coefficient, based on the outer surface A (including fins) is given by,
where the subscript "bank" refers to the external bank-side crossflow, and the subscript "tube" refers to the internal tube-side longitudinal flow. The terms on the right-hand side of Equation (22) may be regarded as resistances to the flow of heat in the presence of a temperature difference. Ai is the total area for heat transfer on the inner tube surface, Ef bank and Ef tube are fin-surface-effectiveness correction factors for externally and internally-finned tube surfaces (equal to unity for plain tubes), and Rf bank and Rf tube are fouling factors which may be estimated using the recommendations in the Standards of the Tubular Exchanger Manufacturers Association, TEMA (1988). Rw is the tube-wall resistance which for plain tubes, of length L, is given by,

where Di is the inner diameter, and λw is the wall thermal conductivity. For finned tubes, Rw is configuration-dependent.
Commonly-employed heat exchanger design methods are the LMTD-based F-correction-factor method, the P-NTU method and the θ method. For the F-correction method, ATLM, is defined by Equation (12), but with ΔTin and ΔTout the temperature differences between the two working fluids at the inlet and outlet. Heat transfer is reduced by a factor F, which is a function of the capacitance ratio, R, and the thermal effectiveness, P, as defined in Table 2, which also gives with the modified rate equation used to calculate
 . The P-NTU method presumes the thermal effectiveness, P (similar to E), to be a function of R, as well as the number of transfer units, NTU = AU/Ccold, of the cold fluid. For the third method, θ = θ(R, NTUcold) but a modified energy balance, instead of a modified rate equation (see Table 2) is used to compute
. The P-NTU method presumes the thermal effectiveness, P (similar to E), to be a function of R, as well as the number of transfer units, NTU = AU/Ccold, of the cold fluid. For the third method, θ = θ(R, NTUcold) but a modified energy balance, instead of a modified rate equation (see Table 2) is used to compute
 . Charts of F, P, and θ may be found in standard references on heat exchangers, often in combined form [see Taborek, Heat Exchanger Design Handbook (1983)]. Regardless of which method is used, two
. Charts of F, P, and θ may be found in standard references on heat exchangers, often in combined form [see Taborek, Heat Exchanger Design Handbook (1983)]. Regardless of which method is used, two
 correlations are needed to calculate U: one, as per Equation (5), to calculate the bank-side crossflow heat transfer coefficient,
correlations are needed to calculate U: one, as per Equation (5), to calculate the bank-side crossflow heat transfer coefficient,
 , the other to calculate the internal tube-side heat transfer coefficient,
, the other to calculate the internal tube-side heat transfer coefficient,
 (See Tubes Single-Phase Heat Transfer in). The temperatures at the solid-fluid interfaces, Tw bank and Tw tube, are obtained as a linear combination of TM bank and TM tube, using the ratio of the resistances in Equation (22) as weighting factors. Tw may be taken as being the temperature between the fluid and the fouling resistance. A typical calculation would then proceed as follows:
(See Tubes Single-Phase Heat Transfer in). The temperatures at the solid-fluid interfaces, Tw bank and Tw tube, are obtained as a linear combination of TM bank and TM tube, using the ratio of the resistances in Equation (22) as weighting factors. Tw may be taken as being the temperature between the fluid and the fouling resistance. A typical calculation would then proceed as follows:
Given Tin bank and Tin tube, guess values for Tom bank, Tout tube, Tw bank, Tw tube (unless known).
Calculate Re and Pr for tube-side and bank-side fluids at the arithmetic-mean bulk temperatures, and values of Prw for the two fluids based on Tw bank and Tw tube.
Compute
 and
and
 using the appropriate correlations applying corrections k1, k2 as necessary, to obtain
using the appropriate correlations applying corrections k1, k2 as necessary, to obtain
 and
and
 . Calculate the overall heat transfer coefficient, U, Equation (22).
. Calculate the overall heat transfer coefficient, U, Equation (22).
Compute the overall rate of heat transfer,
 : Either calculate P and R based on the guessed values and obtain F(P,R) or compute R and NTUc to obtain P(R,NTUc) or θ(R,NTUc) from a chart. Calculate
: Either calculate P and R based on the guessed values and obtain F(P,R) or compute R and NTUc to obtain P(R,NTUc) or θ(R,NTUc) from a chart. Calculate
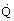 from the formulae in Table II.
from the formulae in Table II.
Compute Tout bank and Tom tube from
 , by means of heat balances applied to both tube-side and bank-side fluids Equation 8. Hence calculate Tw bank and Tw tube (a single value, Tw, will often suffice).
, by means of heat balances applied to both tube-side and bank-side fluids Equation 8. Hence calculate Tw bank and Tw tube (a single value, Tw, will often suffice).
Re-iterate steps 2–5 based on the new values of Tout bank, Tout tube (if necessary) until satisfactory convergence is obtained.
The choice of whether to use the F-correction, P-NTU, or θ methods will depend on the application and may result in substantial simplifications to the general procedure detailed above. If all temperatures are known, the F-correction method can be used with properties enumerated at TM. Conversely, if fluid property variations are negligible and upstream values may be used, the P-NTU and θ methods can be used advantageously. If property variations are significant, but small enough to be considered perturbations about a mean, all the above lumped-parameter-type schemes will necessarily be iterative. For large variations in TM, a numerical scheme is preferred.
While traditional methods are meritorious, the majority of heat exchangers are now designed using computer-software. Many approaches are possible; for example the E-NTU method may be performed over a discrete number of cells corresponding to one or more cylinders. An alternative procedure is to start from first principles, and solve pairs of equations of the form

on a cell-by-cell basis. If an upwind-difference scheme is used,

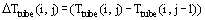
and upstream values are used to ennumerate properties, no iteration is required. Better accuracy can be achieved with a central-difference scheme


Some iteration is now necessary, though by proceeding as indicated in Figure 6, a simple Jacobi point-by-point method will converge rapidly. It is now possible to account for variations of U due to changes in Tb on a cell-by-cell basis. Tw may also be obtained, at an intermediate calculation in each cell to enumerate the dependence of U on Prw. Variations in other properties may be dealt with on a cell-by-cell basis as necessary, depending on the nature of the problem.
The rate equations defined, Equation (24), may be considered like a set of simple enthalpy-conservation equation (convection-source-term equations). It is possible to devise much more sophisticated two-phase enthalpy equations, which account for bank-side mixing, 3D phenomena etc., in addition to bulk convection. It is also possible to generate momentum equations to account for flow-related effects, bypassing, finite-number of rows, etc. For complex flows, the definition of U may be of limited use. Numerical methods differ from traditional methods in that there is no need to rely on the premise that heat transfer is governed by a rate equation. The reader is referred to the articles by D. B. Spalding in the Heat Exchanger Design Handbook (1983), and elsewhere. The use of spreadsheet programs, specialized heat-exchanger-design programs and general-purpose computational fluid dynamics software should be considered prior to writing source code.
Simple lumped-parameter type schemes once formed the basis for all practical heat exchanger design. The situation is changing, and the application of computer software is not only increasing in use and range of application, but is also enhancing our understanding of the physics of flow within the passages of heat exchangers.
REFERENCES
Engineering Sciences Data Unit (1973) Convective Heat Transfer During Crossflow of Fluids Over Plain Tube Banks, ESDU Data Item No. 7303J, London, November.
Engineering Sciences Data Unit (1984) Low-fin Staggered Tube Banks: Heat Transfer and Pressure Drop for Turbulent Single Phase Cross Flow, ESDU Data Item No. 84016, London.
Engineering Sciences Data Unit (1986) High-fin Staggered Tube Banks: Heat Transfer and Pressure Drop for Turbulent Single Phase Gas Flow, ESDU Data Item No. 86022, London, 1986.
Heat Exchanger Design Handbook (1983) Vol. 1–4, Hemisphere, New York.
Standards of the Tubular Exchanger Manufacturers Association, 7th edn. (1988) New York.
Stasiuleviĉius, J. and Skrinska, A. (1988) Heat Transfer of Finned Tube Bundles in Crossflow, 1988. A. A. Žukauskas and G. F. Hewitt (Eds.), Hemisphere, New York. DOI: 10.1016/0142-727X(88)90051-3
Žukauskas, A. A. and Ulinskas, R. V. (1988) Heat Transfer in Tube Banks in Crossflow, Hemisphere, New York.
参考文献列表
- Engineering Sciences Data Unit (1973) Convective Heat Transfer During Crossflow of Fluids Over Plain Tube Banks, ESDU Data Item No. 7303J, London, November.
- Engineering Sciences Data Unit (1984) Low-fin Staggered Tube Banks: Heat Transfer and Pressure Drop for Turbulent Single Phase Cross Flow, ESDU Data Item No. 84016, London.
- Engineering Sciences Data Unit (1986) High-fin Staggered Tube Banks: Heat Transfer and Pressure Drop for Turbulent Single Phase Gas Flow, ESDU Data Item No. 86022, London, 1986.
- Heat Exchanger Design Handbook (1983) Vol. 1–4, Hemisphere, New York. DOI: 10.2514/3.60159
- Standards of the Tubular Exchanger Manufacturers Association, 7th edn. (1988) New York.
- Stasiuleviĉius, J. and Skrinska, A. (1988) Heat Transfer of Finned Tube Bundles in Crossflow, 1988. A. A. Žukauskas and G. F. Hewitt (Eds.), Hemisphere, New York. DOI: 10.1016/0142-727X(88)90051-3
- Žukauskas, A. A. and Ulinskas, R. V. (1988) Heat Transfer in Tube Banks in Crossflow, Hemisphere, New York.